views
Finding the Probability of a Single Random Event
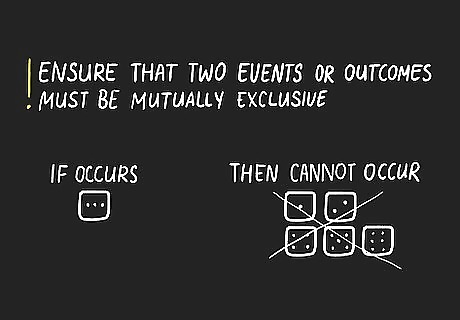
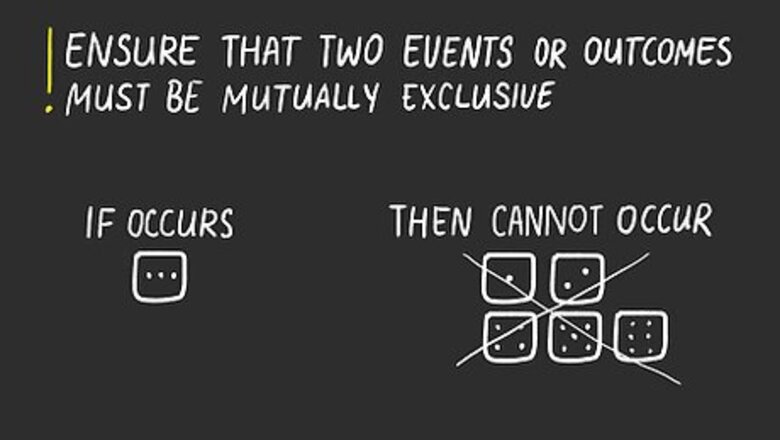
Choose an event with mutually exclusive outcomes. Probability can only be calculated when the event whose probability you’re calculating either happens or doesn’t happen. The event and its opposite both cannot occur at the same time. Rolling a 5 on a die, a certain horse winning a race, are examples of mutually exclusive events. Either a 5 is rolled or it isn’t; either the horse wins or it doesn’t. Example: It would be impossible to calculate the probability of an event phrased as: “Both a 5 and a 6 will come up on a single roll of a die.”
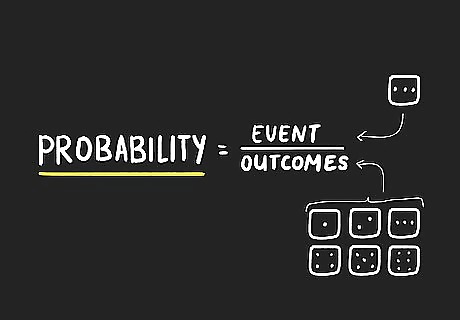
Define all possible events and outcomes that can occur. Let’s say you're trying to find the likelihood of rolling a 3 on a 6-sided die. “Rolling a 3” is the event, and since we know that a 6-sided die can land any one of 6 numbers, the number of outcomes is 6. So, we know that in this case, there are 6 possible events and 1 outcome whose probability we’re interested in calculating. Here are 2 more examples to help you get oriented: Example 1: What is the likelihood of choosing a day that falls on the weekend when randomly picking a day of the week? "Choosing a day that falls on the weekend" is our event, and the number of outcomes is the total number of days in a week: 7. Example 2: A jar contains 4 blue marbles, 5 red marbles and 11 white marbles. If a marble is drawn from the jar at random, what is the probability that this marble is red? "Choosing a red marble" is our event, and the number of outcomes is the total number of marbles in the jar, 20.
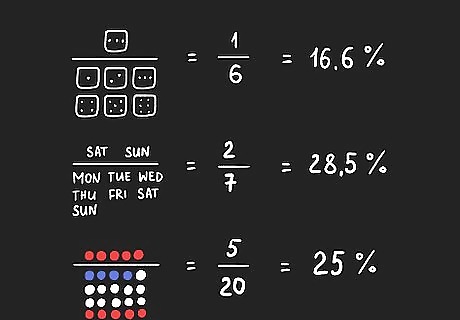
Divide the number of events by the number of possible outcomes. This will give us the probability of a single event occurring. In the case of rolling a 3 on a die, the number of events is 1 (there’s only a single 3 on each die), and the number of outcomes is 6. You can also express this relationship as 1 ÷ 6, 1/6, 0.166, or 16.6%. Here's how you find the probability of our remaining examples: Example 1: What is the likelihood of choosing a day that falls on the weekend when randomly picking a day of the week? The number of events is 2 (since 2 days out of the week are weekends), and the number of outcomes is 7. The probability is 2 ÷ 7 = 2/7. You could also express this as 0.285 or 28.5%. Example 2: A jar contains 4 blue marbles, 5 red marbles and 11 white marbles. If a marble is drawn from the jar at random, what is the probability that this marble is red? The number of events is 5 (since there are 5 red marbles), and the number of outcomes is 20. The probability is 5 ÷ 20 = 1/4. You could also express this as 0.25 or 25%.
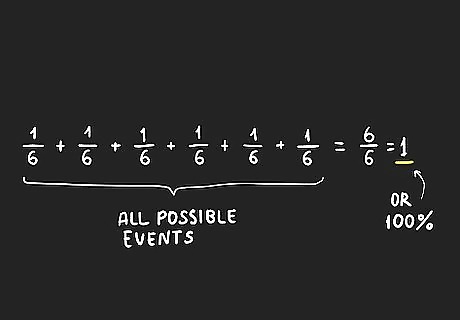
Add up all possible event likelihoods to make sure they equal 1. The likelihood of all possible events needs to add up to 1 or to 100%. If the likelihood of all possible events doesn't add up to 100%, you've most likely made a mistake because you've left out a possible event. Recheck your math to make sure you’re not omitting any possible outcomes. Note: If you had, for example, forgotten about the number 4 on the dice, adding up the probabilities would only reach 5/6 or 83%, indicating a problem. For example, the likelihood of rolling a 3 on a 6-sided die is 1/6. But the probability of rolling all five other numbers on a die is also 1/6. 1/6 + 1/6 + 1/6 + 1/6 + 1/6 + 1/6 = 6/6 , which = 100%.

Represent the probability of an impossible outcome with a 0. This just means that there is no chance of an event happening, and occurs anytime you deal with an event that simply cannot happen. While calculating a 0 probability is not likely, it’s not impossible either. For example, if you were to calculate the probability of the Easter holiday falling on a Monday in the year 2020, the probability would be 0 because Easter is always on a Sunday.
Calculating the Probability of Multiple Random Events
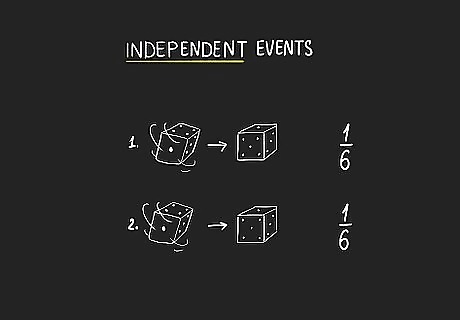
Deal with each probability separately to calculate independent events. Once you’ve figured out what these probabilities are, you’ll calculate them separately. Say you wanted to know the probability of rolling a 5 twice consecutively on a 6-sided die. You know that the probability of rolling one five is 1/6, and the probability of rolling another five with the same die is also 1/6. The first outcome doesn’t interfere with the second. Note: The probability of the 5s being rolled are called independent events, because what you roll the first time does not affect what happens the second time.
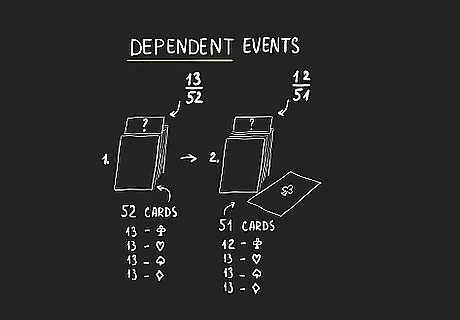
Consider the effect of prior events when calculating probability for dependent events. If the occurrence of 1 event alters the probability of a second event occurring, you are measuring the probability of dependent events. For example, if you choose 2 cards out of a deck of 52 cards, when you choose the first card, that affects what cards are available when you choose the second card. To calculate the probability for the second of two dependent events, you’ll need to subtract 1 from the possible number of outcomes when calculating the probability of the second event. Example 1: Consider the event: Two cards are drawn randomly from a deck of cards. What is the likelihood that both cards are clubs? The likelihood that the first card is a club is 13/52, or 1/4. (There are 13 clubs in every deck of cards.) Now, the likelihood that the second card is a club is 12/51, since 1 club will have already been removed. This is because what you do the first time affects the second. If you draw a 3 of clubs and don't put it back, there will be one less club and one less card in the deck (51 instead of 52). Example 2: A jar contains 4 blue marbles, 5 red marbles, and 11 white marbles. If 3 marbles are drawn from the jar at random, what is the probability that the first marble is red, the second marble is blue, and the third is white? The probability that the first marble is red is 5/20, or 1/4. The probability of the second marble being blue is 4/19, since we have 1 less marble, but not 1 less blue marble. And the probability that the third marble is white is 11/18, because we’ve already chosen 2 marbles.
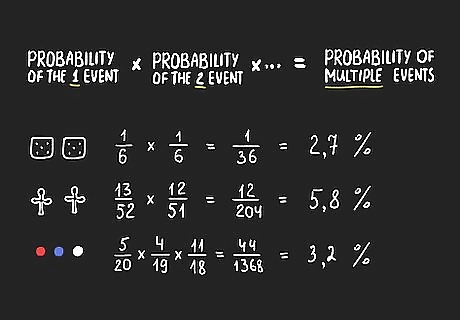
Multiply the probabilities of each separate event by one another. Regardless of whether you’re dealing with independent or dependent events, and whether you’re working with 2, 3, or even 10 total outcomes, you can calculate the total probability by multiplying the events’ separate probabilities by one another. This will give you the probability of multiple events occurring one after another. So, for the scenario; What is the probability of rolling two consecutive fives on a six-sided die? the probability of both independent events is 1/6. This gives us 1/6 x 1/6 = 1/36. You could also express this as 0.027 or 2.7%. Example 1: Two cards are drawn randomly from a deck of cards. What is the likelihood that both cards are clubs? The probability of the first event happening is 13/52. The probability of the second event happening is 12/51. The probability is 13/52 x 12/51 = 12/204 = 1/17. You could also express this as 0.058 or 5.8%. Example 2: A jar contains 4 blue marbles, 5 red marbles and 11 white marbles. If three marbles are drawn from the jar at random, what is the probability that the first marble is red, the second marble is blue, and the third is white? The probability of the first event is 5/20. The probability of the second event is 4/19. And the probability of the third event is 11/18. The probability is 5/20 x 4/19 x 11/18 = 44/1368 = 0.032. You could also express this as 3.2%.
Converting Odds to Probabilities

Set the odds as a ratio with the positive outcome as a numerator. For example, let’s return to our example dealing with colored marbles. Say you want to figure out the probability of drawing a white marble (of which there are 11) out of the total pot of marbles (which contains 20). The odds of the event happening is the ratio of the probability that it will occur over the probability that it will not occur. Since there are 11 white and 9 non-white marbles, you’ll write the odds as the ratio 11:9. The number 11 represents the likelihood of choosing a white marble and the number 9 represents the likelihood of choosing a marble of a different color. So, odds are that you will draw a white marble.
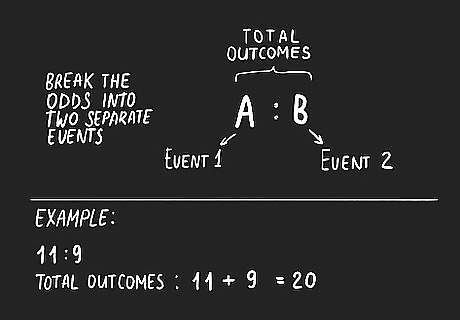
Add the numbers together to convert the odds to probability. Converting odds is pretty simple. First ,break the odds into 2 separate events: the odds of drawing a white marble (11) and the odds of drawing a marble of a different color (9). Add the numbers together to calculate the number of total outcomes. Write this as a probability, with the newly calculated total number of outcomes as the denominator. The event that you’ll draw a white marble is 11; the event another color will be drawn is 9. The total number of outcomes is 11 + 9, or 20.
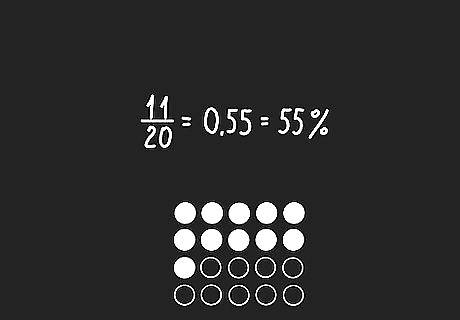
Find the odds as if you were calculating the probability of a single event. You have calculated that there are a total of 20 possibilities and that, essentially, 11 of those outcomes are drawing a white marble. So, the probability of drawing a white marble can now be approached like any other single-event probability calculation. Divide 11 (number of positive outcomes) by 20 (number of total events) to get the probability. So, in our example, the probability of drawing a white marble is 11/20. Divide this out: 11 ÷ 20 = 0.55 or 55%.

















Comments
0 comment